JEE 2015 :: Main 2015 :: Physics 2015

• Main 2015 - Physics 2015
• Main 2015 - Chemistry 2015
• Main 2015 - Mathematics 2015

A solid body of constant heat capacity 1 j/^oC is being heated by keeping it in contact with reservoirs in two ways

(i) Sequentially keeping in contact with 2 reservoirs such that each reservoir supplies the same amount of heat.

(ii) Sequentially keeping in contact with 8 reservoirs such that each reservoir supplies the same amount of heat.

In both the cases, body is brought from initial temperature 100^oC to final temperature 200^oC. Entropy change of the body in the two cases respectively , is

Answer:

Explanation:

Since entropy is a state function, therefore change in entropy in both the processes must be same. Therefore,correct option should be ln2, ln2

From a solid sphere of mass M and radius R ,a cube of maximum possible volume is cut.Moment of inertia of cube about an axis passing through its centre and perpendicular to one of its face is 

Answer:

Explanation:

Central idea:

Use geometry of the figure to calculate mass and side length of the cube interns of M and R respectively.

Consider the cross-sectional view of a diametric plane as shown in the adjacent diagram.

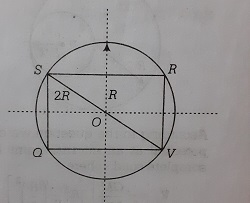
Cross-sectional view of the cube and sphere

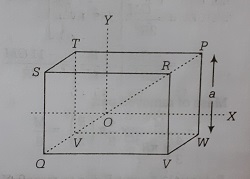
Using geometry of the cube

$PQ=2R=(\sqrt{3}a)$ or $a=\frac{2R}{\sqrt{3}}$

Volume density of the solid sphere

$\rho=\frac{M}{\frac{4}{3}\pi R^{3}}=\frac{3}{4\pi}\left(\frac{M}{R^{3}}\right)$

Mass of cube $(m)=(\rho)(a)^{3}$

$=\left(\frac{3}{4\pi}\times \frac{M}{R^{3}}\right)$

$=\left(\frac{3}{4\pi}\times \frac{M}{R^{3}}\right)\left[\frac{2R}{\sqrt{3}}\right]^{3}$

$=\frac{3M}{4\pi R^{3}}\times \frac{8R^{3}}{3\sqrt{3}}$

$\frac{2M}{\sqrt{3}\pi}$

Moment of interia of the cube about given axis is

$=\frac{3M}{4\pi R^{3}}\times \frac{8R^{3}}{3\sqrt{3}}$

$I_{y}\frac{ma^{2}}{6}=\frac{2M}{\sqrt{3}\pi}\times \frac{1}{6}\times \frac{4R^{2}}{3}=\frac{4MR^{2}}{9\sqrt{3}\pi}$

Distance of centre of mass of a solid uniform cone from its vertex is z₀.If the radius of its base is R and its height is h, then z₀ is equal to

Answer:

Explanation:

We know that centre of mass of a uniform solid cone of height h is at height h/4 from base ,therefore 

$h-z_{0}=\frac{h}{4}$

$z_{0}=h-\frac{h}{4}=\frac{3h}{4}$

A particle of mass m moving in the x-direction with speed 2v is hit by another particle of mass 2m moving in the y-direction with speed v .If the collosion is perfectly inelastic the percentage loss in the eneergy during the collosion is close to

Answer:

Explanation:

Central Idea: Conservation of linear momentum can be applied but energy is not conserved.Consider the movement of two particles as shown below.

Conserving linear momentum in x-direction 

(p_i)x=(p_f)x

or 2mv=(2m+m)v_x

v_x=$\frac{2}{3}v$

Considering linear momentum in y-direction

(p_i)y=(p_f)y

or 2mv=(2m+m)vy

V_y=$\frac{2}{3}v$

Initial kinetic energy of two particles sysytem is 

$E_{i}=\frac{1}{2}m(2v^{2})+\frac{1}{2}(2m)(v)^{2}$

$=\frac{1}{2}4mv^{2}+\frac{1}{2}2mv^{2}$

$3mv^{2}$

Final energy of the combined two particles system is 

$E_{f}=\frac{1}{2}(3m)(v_x^2+v_y^2)$

$=\frac{1}{2}(3m)\left[\frac{4v^{2}}{9}+\frac{4v^{2}}{9}\right]$

$=\frac{3m}{2}\left[\frac{8v^{2}}{9}\right]=\frac{4mv^{2}}{3}$

loss in the energy 

$\Delta E=E_{i}-E_{f}$

$mv^{2}\left[3-\frac{4}{3}\right]=\frac{5}{3}mv^{2}$

Percentage loss in the energy during the collision

$\frac{\Delta E}{Ei}\times 100=\frac{\frac{5}{3}mv^{2}}{3mv^{2}}\times 100=\frac{5}{9}\times 100$

$\simeq 56%$

The period of oscillation of a simple pendulam is $T= 2\pi \sqrt{\frac{L}{g}}$.Measured value of L is 20.0 cm known to 1 mm accuracy and time for 100 oscillation  of the pendulam is found to be 90 s using  a wrist watch of 1 sresolution .The accurancy in the determination of g is

Answer:

Explanation:

Central idea :Given time period $T= 2\pi \sqrt{\frac{L}{g}}$ 

Thus,Changes can be expressed as 

$\pm \frac{2\Delta T}{T}=\pm \frac{\Delta L}{L}\pm \frac{\Delta g}{g}$

According to the question, we can write

$\frac{\Delta L}{L}=\frac{0.1 cm}{20.0 cm}= \frac{1}{200}$

Again time period

$T= \frac{90}{100} s $and $\Delta T=\frac{1}{100} s$

$\frac{\Delta  T}{T}=\frac{1}{90} s$

Now,

$T=2\pi \sqrt{\frac{L}{g}}$

$g=4\pi ^{2}\frac{L}{T^{2}}$

$\frac{\Delta g}{g}=\frac{\Delta L}{L} + \frac{2\Delta T}{T}$

$\frac{\Delta g}{g}\times 100%=\frac{\Delta L}{L}\times 100% + \frac{2\Delta T}{T}\times 100%$

$=\left ( \frac{1}{200}\times 100 \right )% + 2\times \frac{1}{90}\times 100% $

$\simeq2.72 \simeq 3%$

• Main 2015 - Physics 2015
• Main 2015 - Chemistry 2015
• Main 2015 - Mathematics 2015